Return to the clickable list of items

201) A “nuclear reactor“ in a garage?

Ludwik Kowalski (3/7/05)
Department of Mathematical Sciences
Montclair State University, Upper Montclair, NJ, 07043

In the unit 170 I wrote: “. . . Another thing that impressed me was that one of the teams that confirmed Naudin’s findings consisted of highly trained electrochemist. On the other hand, I was slightly disappointed that the identity of the US laboratory, in which the confirming calorimetric experiments were conducted, was not specified. The document shows the photo of two researchers from Louisiana, [see below] and their first names (two nice looking young girls, probably students), but not the name of the laboratory, or the name of the project director. Was it a high school project or a projects at a well known university? Such information is important when controversial results are presented. Why was it not provided by Naudin?”

These students, X and Y, prefer to remain anonymous (see below).

Two weeks ago one of these girls, Y, sent me an email message. She wrote: “This project was not done at a laboratory. This was a Science Fair Project that Y and I did last year. The picture on Naudin’s website was actually taken at the 2004 International Science and Engineering Fair in Portland, OR. This was a team project. Y, being a senior, has now graduated, and I am continuing the project this year with the following changes:

1) A much more accurate scale, calibration checked at a local chemical plant lab.
2) Using Potassium carbonate rather than Sodium Hydrogen carbonate.
3) A completely sealed system to condense the steam and calculate the Hydrogen generated.
4) A new reactor (thermos design. mist eliminator, stainless internal used as the anode
5) Digital thermometer calibrated with distilled ice and boiling water
6) Input power readings taken with digital watt meter every 15 seconds.

. . . Last year our best was 147%, with an average of 117%, including our learning curve. This year the average is 143%, with the best being 271%. Being a senior, this is now my last year….but if I were to continue further, I would try the experiment under higher pressure – like 100 psi or so, to look for improvements.

This work was not done at a lab – it was done at my house, in the garage. Most of the items needed to build the power supply were purchased on eBay… Sounds dangerous….two high school girls build a nuclear reactor in their back yard… Finally, should anyone write to you for advice about a cold fusion project, prepare them for the amount of skepticism that they will be subject to with that kind of work. At Internationals, the worst thing we heard was from what we thought would have been the most educated judges…but they were not very accepting. More than once we heard “If it would have worked, you would be dead.

Y
Senior,Catholic High School
New Iberia, Louisiana

It is good to know that the spirit of scientific and technological adventure did not disappear; I am thinking about Marie Curie and Thomas Edison. But I am concerned about safety; the power supply they have (200 volts and up to 10 A) can easily kill a person. Such experiments should be conducted in school laboratories and be supervised by knowledgeable teachers. I sent Y a description of a recent accident with a similar setup in Japan (Mizuno’s lab) but she did not comment.

Responding to the above I wrote (on 2/7/05):
1) I was very happy to receive your message; thanks for contacting me. I am also glad that you continue working on the project. But are you taking appropriate precautions in working with a very dangerous electrical power supply, and with hazardous chemicals? Is your work supervised by a trained person? I hope your teacher (or teachers) are there to prevent bad things from happening in the garage. Did you ask for permission to conduct the experiment at school?

2) I am not a chemist. Can you explain to me why are you changing an electrolyte that was delivering positive results?

3) I would not worry about the limited accuracy (0.2%) of the watt-meter when the average excess power is 43%.

4) The big challenge in this field is to convince others, including teachers and competition judges, that the anomaly is real. This is a very difficult task, considering the effects of negative publicity.

5) After retiring last year I decided to find an experiment that would be convincing to all honest skeptics. For that reason I contacted Dr. Richard Oriani (a retired chemist from the University of Minnesota) and we are working together now. The goal is to develop a simple setup that gives 100% reproducible results. Oriani’s electrolytic cell is much smaller than yours; it is receiving electric energy at the rate of several watts. That is sufficient to observe nuclear particles; we are not focusing on excess heat, as you are.

6) After visiting Oriani, to work with him for one week. After that I performed ten experiments in my own home. Unfortunately, the goal of 100% reproducibility (ability to get an observable effect from each experiment in any laboratory) has not yet been achieved. But we are not giving up; this work is in progress.

7) I do believe that your excess heat, and excess heat in Naudin’s experiments, are real. But that is not the main issue. The issue with all these experiments, as far as I can tell, is absence of evidence that the origin of excess heat is nuclear. One has to perform a complete analysis of all reactions to rule out chemical origin of excess heat. Neither Naudin nor you performed such analysis, as far as I know. Oriani also worked on excess heat experiments but decided (for the time being, I suppose) to focus on nuclear particles.

8) When will your next experiment be performed? I would be happy to send you several detectors of nuclear particles, if you want. They are small plastic chips (very clean polycarbonate) to be placed above the electrolyte, and in the electrolyte. After the experiment you would send the chips back to me and I would analyze them. If we see particles then we will try to repeat the experiment several times. Perhaps your large cell will become a simple setup needed to convince honest skeptics. If so we will try to publish these results in a mainstream journal.

9) Where is X now? Are you still collaborating on this project? What do you plan to do after the graduation?

That is how our correspondence started. Y will apply the chips to the electrolyte and to the electrodes this Sunday. The exposure will be three days. As usual, I want to be sure that nothing is radioactive before the main test. While waiting let me describe her setup. It is Naudin’s “cold fusion reactor” with some modifications. Imagine a beaker with two electrodes, for example, both made of tungsten. The current flowing through the electrolyte, between 5 and 10 A, is sustained by a d.c. power supply of about 200 V. Intensive arcing and sparking is taking place in the liquid and the cathode is consumed rapidly, for example, after five to ten minutes. The claim is that the electric energy supplied to the cell, Ee, is smaller that the energy released in the cell, Et. The difference, Ex=Et-Ee, is called excess energy; it is mostly thermal. The ratio of Et/Ee, is called efficiency.

The average efficiency reported by Y (see above) was 1.47. It means that for every 1,000 J of electric energy the cell releases 1,470 joules of heat. The origin of Ex (470 J) is assumed to be nuclear but no evidence for this is provided. The CR-39 chips will be used by us to explore a possibility that nuclear particles, similar to those discovered by Oriani, are emitted. That could become the needed evidence validating Naudin’s claim. Ignoring that aspect let me focus on details of excess heat measurements. The value of Ee is determined by Y as the sum of many P*dT terms, where P are power readings of a watt-meter and dT is the time interval (15 seconds) between consecutive readings. One of Y’s experiments lasted 3 minutes and the value of Ee, delivered during that time interval, turned out to be 87,780 J.

The efficiency of this experiment was reported as 1.92. This implies that Et was found to be 1.92*1,470 = 2,822 J. How was Et measured? It turns out that Et consists of several components; I will label them as E1, E2, E3, E4, E5, etc. The E1 is the energy needed to increase the temperature of the electrolyte to the boiling point, E2 is the energy used to evaporate m1 grams of water, and E3 is the energy used to decompose m2 grams of water (bubbles of oxygen and hydrogen escaping from the cell). It is well known that 2,260 J are needed to evaporate each gram of water and that 13,174 J are needed to decompose each gram of that liquid. Thus, in the first approximation:

Et = E1+E2+E3 = s*(T2-T1) + 2,260*m1 + 13,174*m2

where T1 is the initial temperature, T2 is the boiling temperature and s is the heat capacity of the calorimetric vessel. The first term, E1, becomes negligible when the electrolyte is preheated before the current is turned on (to make T1=T2). In Y’s experiment, however, (T2-T1) was 44 C and the value of s was found to be 116.4 J/C. That mean value of s was determined by Y from eleven preliminary experiments.

In many Naudin’s experiments the vessel was an open beaker. Knowing that m2<<m1 (ignoring E3) Naudin measures the mass of the vessel before and after the electrolysis. The difference, identified as m1, was then used to calculate E2. An interesting innovation, introduced by Y, was to determine m1 and m2 separately. (In Naudin’s experiments water vapor, oxygen and hydrogen escape into air and the measured mass difference is actually the sum of m1 and m2). I will return to this subject a little later. Using the above two equations one has:

Ex = (E1+E2+E3+E4) - Ee = [s*(T2-T1) + 2,260*m1 + 13,174*m2 + E4] - Ee

In this equation E4 represents the amount of thermal energy lost by conduction and convection during the electrolysis. It is interesting to note that by ignoring E4 one makes a systematic error of underestimation of Ex. In other words, the values of Ex reported by those who ignore E4 are smaller than the true values. Y’s calorimetric vessel is a nearly sealed thermos, as illustrated in Figure 2. In that way the value of E4 is reduced significantly, in comparison with what it would be in an open beaker.

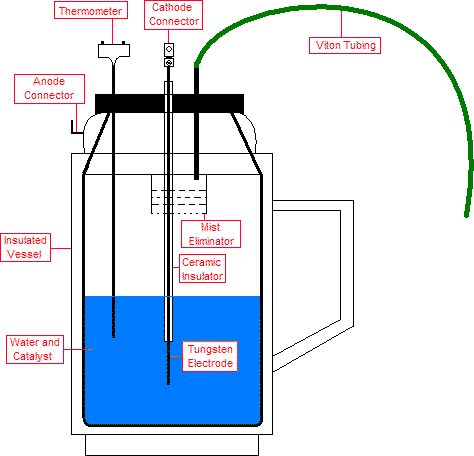
Sealed thermos. The green tube connects to the condenser below.

Condenser; the top is exposed to the atmosphere.

The vapor and gasses escape from the thermos through a flexible tube connected to a second container. That container, called condenser, is filled with cold water. The exit from the flexible pipe is under cold water. Bubbles of hydrogen and oxygen escaping from the exit are released into the atmosphere. The water vapor, on the other hand, condenses and remains in the container. Both vessels, the thermos and the condenser, are standing of sensitive electronic scales. The change in the mass of the condenser is interpreted as m1 while the change in the mass of the thermos is interpreted as m1+m2. That is how the values of m1 and m2 are measured by Y. In the experiments in which the efficiency was 1.92 the values of m1 turned out to be 35.7 grams while the value of m2 turned out to be 0.6 grams. Showing that m2 is indeed much smaller than m1 has a great pedagogical value; most students are not aware of that fact.

Y also wrote to me about another complication. It has to do with vapors that condense in the thermos, for example, on its walls and near the exit to the flexible pipe. Suppose that 5% of vapor condenses inside the thermos. This has two important consequences: (a) the value of E2 is underestimated by 5% and (b) energy released through condensation remains in the thermos. Y tried to minimize condensation of vapors inside the cell but I am not convinced that she was successful. She wrote: “If you look at the attached picture you'll see a "sink strainer" where the insulator comes thru the thermos top. This is actually two sink strainers glued together with JB Weld epoxy. I got these at Wal-Mart. Between the two strainers I have three layers of 100 mesh stainless steel screen.

Once I put the preheated solution into the thermos and close it up, I let it set for a while for everything to come to an even temperature. This takes about a minute or so for the temp to level out. I ASSUME the mist eliminator is about up to temperature for very little condensation to occur at this point in the vessel ... although I'm sure there's some dripping back into the bottom of the reactor from here.”

If I were asked to measure the Ex accurately I would not use a condenser. My setup would consist of two open cells, as illustrated below. The left beaker contains the electrolyte, the right beaker contains the same amount of distilled water and an ohmic rheostat immersed in it. The current in the left cell is ionic while the current in the right cell is electronic. The variable resistance of the rheostate would be chosen to make the two currents equal. To impose the right side current of 10 A at 200 V, for example, the resistance would have to be 20 ohms. To change the current to 5 A, also at 200 V, the resistance would have to be increased to 40 ohms. Note that the applied voltages are essentially identical because cells are connected to the same power supply. It would not be difficult to adjust the reostate manually during an experiment lasting several minutes. One would watch the current of the left ammeter and change the resistance, by as much as necessary, to match it with the current through the right ammeter. In that way the Ee would be identical in two cells.

A schematic diagram of a setup with two cells.

The sizes, of two cells, their masses, and their thermal capacities would be essentially identical. It is well known that a current flowing through an ohmic resistor (in the right cell) generates no excess heat. Therefore, the difference between E2 in the left cell and E2 in the right cell, if any, would be eqaul to Ex. Note that absence of information about E1, E3 and E4 would not prevent me from the determination of Ex. The same idea could be implemented with two cells connected in series. In that case I would use one ammeter and two voltmeters, one for each cell. The reostate would be adjusted to keep two voltages identical (to make sure that Ee are identical).

Let me end this essay by saying that I was highly impressed by what the two high school girls did in a Louisiana garage. It is a pity that “educated judges” were so skeptical when Y and X presented their outstanding work at an international competition. The judges probably thought that the project was pseudoscientific and not worth their attention. I would expect them to focus on what was positive in this explorative work. What is wrong with a student project trying to prove, or disprove, a controversial claim? What can be a better way to expose students to the excitement of scientific research than working on such projects? I am glad that Y decided to contact me and excited about our pending investigation of a “nuclear signature” in her setup. Information about that investigation is going to be appended below.

Appended on 2/18/05:
To concentrate on condensation inside the vessel let me consider a hypothetical example. An open jar, containing an electrolyte, stands on a scale; in that way the total mass can be measured at any time. The electrolyte is brought to the boiling temperature (by warming it on a hot plate or by passing an electric current through it). The first measurement of P is made at an arbitrary moment after the boilimg started. Consecutive values of P are recorded avery 15 seconds, as in Y’s experiment. Suppose that the current was turned off after 5 minutes. The amount of water, m, lost during that time was 50 grams. The value of Ee, calculated from consecutive recordings of P, was 80,000 J. Ignoring the mass the escaping hydrogen and oxygen the value of Et was calculated as 2260*50=113,000 J. The excess heat, and the efficiency, are then calculated as Ex = 113,000 - 80,000 = 33,000 J and ef=113,000/80,000=1.41, respectively.

The jar was not transparent and I assumed, in the above calculation, that no vapor condensed on the walls during the electrolysis. Suppose somebody tells me that 10 grams of vapor condensed and returned to the boiling electrolyte. My task is to recalculate the Ex by taking this into account. The first step is simple; I change the evaporated mass from 50 to 60 grams. The corrected value of Et becomes 2260*60 = 135,600 J. The difference between this value and Ee is 55,600 J (instead of 33,000 J above). This is 22,600 J larger than above. The reason for this is that thermal energy is released in condensation. The latent heat of condensation is the same as latent heat of evaporation. This means the 2260*10=22,600 j of thermal energy was released.

Does it mean that the corrected value of Ex should be 135,600 - 80,000 = 55,600 J? Not necessarily. That answer would be correct only if the thermal energy released through condensation went entirely to the surrounding air. That is one possible extreme. The other extreme is when all the released energy goes back to the electrolyte. In that case the Ex would be 135,600 - 80,000 +22,600 = 78,200 J. Why was the condensation energy added and not subtracted? Because that energy, like Ee, enters the system. In reality, however, only a fraction of 22,660 J enters the system; the remaining part goes into the air. Suppose that the unknown fractions are 50% each. In that case Ex would be 567,300 J. Unfortunately, it is very difficult to determine how the released energy is divided between the outside air and the inside electrolyte. This shows how much uncertainty is introduced into the calculation of Ex when condensations of vapors takes place inside the electrolytic cell. I think that Y recognized this fact and tried to reduce the uncertainties by using a condenser.

Appended on 2/19/05:
It is reassuring to know that by ignoring condensation of vapor inside the electrolytic cell, like ignoring heat losses due to conduction and convection, leads to an underestimation, not to an overestimation, of excess heat. The values reported by Naudin and others are most likely smaller than the true values of excess heat. But what is the origin of that heat? That is still an open question.

In most excess heat research Ex is generated at the rates of watts or fractions of watts. In Naudin-like experiments, on other hand, Ex is generated at the level of hundreds of watts. Tracing chemical reactions taking place in high power cells is likely to be more difficult than in low power cells. Nuclear signatures, by contrast, might be proportional to the cell power. If this is true then discovering nuclear particles in high power cells might be easier.

Appneded on 3/1/05:
Y sent me back the CR-39 chip that were exposed (for three days) to her electrodes and her electrolyte. These electrodes were to be used in our anticipated experiment. The chip exposed to the tungsten cathode had a lot of tracks. I suspect that tungsten rods are welding electrodes containing about 2% of thorium. Such electrodes were widely used in the past, probably to ionize air. The chip that was exposed to tungsten had thousands of tracks, even more than my control chip exposed to an alpha source. Informing Y about this finding I wrote: “Your tungsten has alpha-radioactive material in it; you can probably detect it with a Geiger counter in your school. I suspect it is thorium or uranium. We can not go ahead with the suggested experiment, unless you can use another cathode. Let me know.” In the next message I added: “In reading Naudin's descriptions again I see that stainless steel could be used as a cathode. I would be surprised to find a radioactive material in a spoon or a knife made from this alloy. But the new material would have to be checked, if you decide to continue working on our project.”

Appended on 3/7/05:
This web page contained true names of students. But I did not want to share this information over the Internet without asking for permission. I am glad I asked. The reply, received this morning, was: “We would prefer to keep our last names out of the reports. You never know these days”. That explains why the real names were replaced with X and Y. Additional information about this project will be appended, if Y decides to continue.

Return to the clickable list of items